lambda
Opening Days 3
Announcements
- Welcome to IDS-101-13: Thinking Machines!
- Opening Days: Day 3... Do you know where to procure?
- Over-the-counter medication
- Prescription medication
- A COVID vaccine
- Specialty foods, like electrolyte or meal-replacers
- How did the Pythagorean Triples homework go?
- Some parts were intended to be obnoxious.
- I hope you were rigorous around
- Text formatting
- Recording your thoughts
- Collaborating
- Mostly because it's good practice.
- Today's lecture loosely adapted from here but in a nonobvious way so maybe stay away from that link for now.
Python
- This semester we will use Python
- Python is a language, but a novel kind of language.
- Python is free,
- Python is very widely used,
- Python is flexible,
- Python is relatively easy to learn,
- and Python is quite powerful.
- It may be the case that Python can be thought of as the language in which a machine may think.
- Deliberately weak claim.
Pythogoras
- Let's review the homework
- If I were you I would:
- Leave my original homework unaltered.
- Append anything you learn today to the end.
- Thereby preserve a record you can look back on.
- Some elements of the homework were "deliberately annoying"
- We want to introduce "problems" or even just annoyances before we introduce their solutions.
- This is supported by some educational literature - you'll just have to let me know what you think.
- If I were you I would:
Review:
- Homework 1 Pythagorean Triples
- Write code to define positive integers a, b, and c of your own choosing.
- A few solutions.
- Multi-line, multi-cell.
- Create 3 "Code" blocks.
- Assign one variable in each.
a = 3
b = 4
c = 5
- A good way to make quick changes to individual variables.
- Cell structure is more complicated.
- Multi-line, multi-cell.
Review:
- Homework 1 Pythagorean Triples
- Write code to define positive integers a, b, and c of your own choosing.
- A few solutions.
- Multi-line, single-cell.
- Create 1 "Code" block.
- Make three lines, one variable in each.
a = 3 b = 4 c = 5
- A good way to use fewer cells.
- Individual cells are more complex.
- This is the Gemini (AI) solution.
- Multi-line, single-cell.
Review:
- Homework 1 Pythagorean Triples
- Write code to define positive integers a, b, and c of your own choosing.
- A few solutions.
- Single-line
- I haven't shown you this, but I when dealing with a "triple" I like to assign it all at once.
- I use commas to assign 3 variable names to 3 values.
a, b, c = 3, 4, 5
- I was able to get Gemini to do this with the following prompt:
Write code to define positive integers a, b, and c of your own choosing in a single line - This line is harder to read and weirder to edit, but
- There are many fewer lines.
- Single-line
Be sure to introduce yourselves!
| Group 1 | Group 2 | Group 3 | Group 4 |
|---|---|---|---|
Question #1
How do you decide how to express yourself in a constructed language?
- Think about a possible answer on your own
- Discuss your answers with the rest of the group
- Record a summary of each group’s discussion
If your initial answer is e.g. "I just do the first thing I think of" or "I just follow a guide" - dig deeper.
Why is that the first thing you think of?
How do you choose a guide?
How do you decide what prompt to give an AI?
Review:
- Homework 1 Pythagorean Triples
- Calculate a2, b2, and c2
- As with picking values, a few options:
- Multi-cell
- Multi-line / single-cell
- Single-line
- But an addition wrinkle: a2 is not meaningful Python
- This is an example of something that exists in a natural language but not a constructed langage.
- Python, and many other programming languages, often lack to notion of "superscripts" or raised text.
- Natural languages, which may have initially been written by hand on paper, can easily accomodate these features.
Review:
- Homework 1 Pythagorean Triples
- a2 is not meaningful Python
- So what do we do?
- "Squaring" can be thought of, in my mind, in two ways.
- Multiply: I square some value by multipling that value by itself.
a_squared = a * a - Exponentiation: I square some value by raising it to the second power.
a_squared = a ** 2 - I should also verify:
a ** 2 == a * a
- Multiply: I square some value by multipling that value by itself.
Review:
- Homework 1 Pythagorean Triples
- a2 is not meaningful Python
- So what do we do?
- "Squaring" can be thought of, in my mind, in two ways.
- I gravitate toward multiplication.
- In my mind: multiplication is easier that exponentiation, so I'll use that.
- So I could write, perhaps:
a_2, b_2, c_2 = a*a, b*b, c*c - Gemini did something quite different (for me):
# prompt: Calculate a2, b2, and c2 a2 = a**2 b2 = b**2 c2 = c**2
Question #2
What are some reasons humans and machines may choose different ways to solve the same problems?
- Think about a possible answer on your own
- Discuss your answers with the rest of the group
- Record a summary of each group’s discussion
Did you use the same or different solutions than a machine might?
Review:
- Homework 1 Pythagorean Triples
- Check to see if your three values form a Pythagorean Triple such that a2 + b2 = c2.
- Have Python print True or False to verify that you do, or do not, have a Pythagorean Triple defined.
- Hint: You will need to use double and single equals as well as print()
- We use double equals to determine the true/false value of the equality.
a_2 + b_2 = c_2 - We can use single equals again to assign the result to a variable.
is_triple = a_2 + b_2 = c_2 - We can use print() to "print" to true/false
print(is_triple) - We could, of course, do everything in a single line
print(a*a + b*b = c*c)
- We use double equals to determine the true/false value of the equality.
- I asked for print make an important point:
is_triple == print(is_triple) # this is "False"- Printing true/false and determining true/false are different things - it's good to keep this in mind.
Question #3
Reflect on what you learned about
- Python
- Thinking
- Language, and
- Machines
- Discuss your answers with the rest of the group
- Record a summary of each group’s discussion
Fake Functions
- Suppose we wish to check more than one triple.
- We could, for example, have two Code cells:
- In the first, we assign variables:
a, b, c = 3, 4, 5 - In the second, we print:
print(a*a + b*b == c*c) - Then it is simply a matter of changing the variables and "running" two cells.
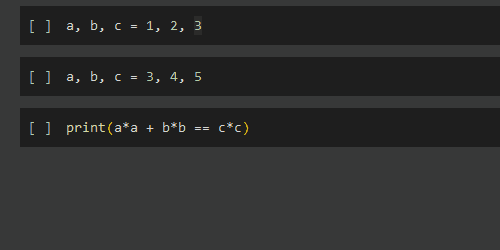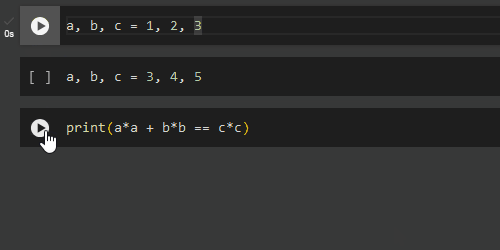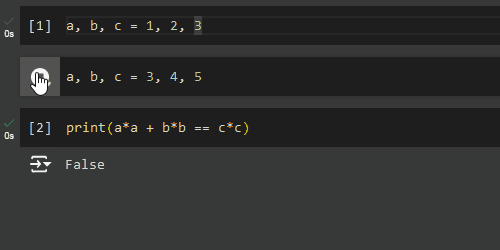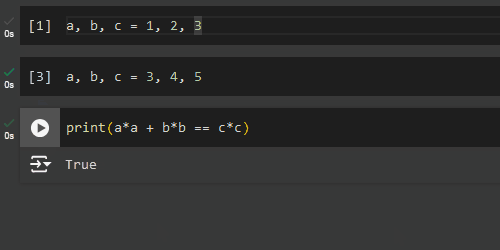
- Essentially, we have created a way to take three numbers and return a true/false value.
- We term this idea a function.
Functions
- Python contains the "lambda" keyword to make functions:
- Let's see it in action.
Functionless Function-ily 
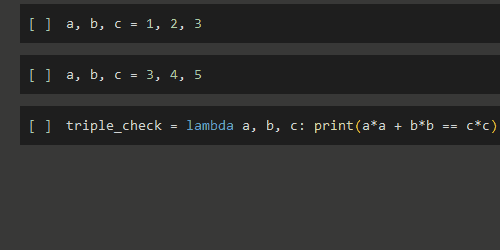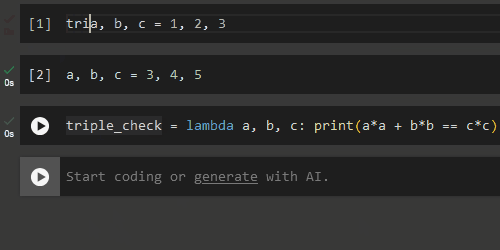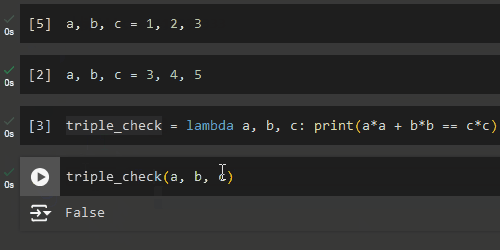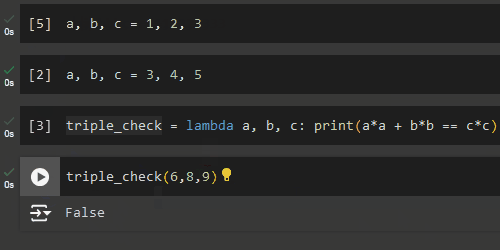
- It looks like this:
triple_check = lambda a, b, c: print(a*a + b*b == c*c) - Now: explore how functions work and can be used.
Recall:
- Exercise A.5 One or two equal signs.
- Select any two integers and assign them to any two variable names, such as "x" or "y"
- It will help latter if the first value is much larger than the second, but you can always change latter.
- Calculate the integer "quotient" (the result of integer divison) and remainder of the two values
- What operations should you use?
- Store the result of each in a variable, for latter
- Use "double equals" to check if
- the smaller value (say, y)
- times the quotient (say, q)
- plus the remainder (say, r)
- is equal to the larger value (say, x)
For Today:
- Exercise A.6 A novel function.
- Write a function that, given two numbers, say "a", "b", prints "True" if:
- the second value (say, y)
- times the quotient of the two values
- plus the modulo of the two values
- is equal to the first of the two values (say, x)
- And prints "False" otherwise.
- Write a function that, given two numbers, say "a", "b", prints "True" if:
Question #4
What are some ways you think or act that feel like functions?
- Think about a possible answer on your own
- Discuss your answers with the rest of the group
- Record a summary of each group’s discussion
In your group, you may also wish to discuss how you feel about functions, Python, Opening Days, etc. Feel free to do so!
No Homework
- Be ready to begin the first week of your other classes!